Behind the numbers: What does a COVID-19 ‘positivity rate’ really mean?

Go Deeper.
Create an account or log in to save stories.
Like this?
Thanks for liking this story! We have added it to a list of your favorite stories.
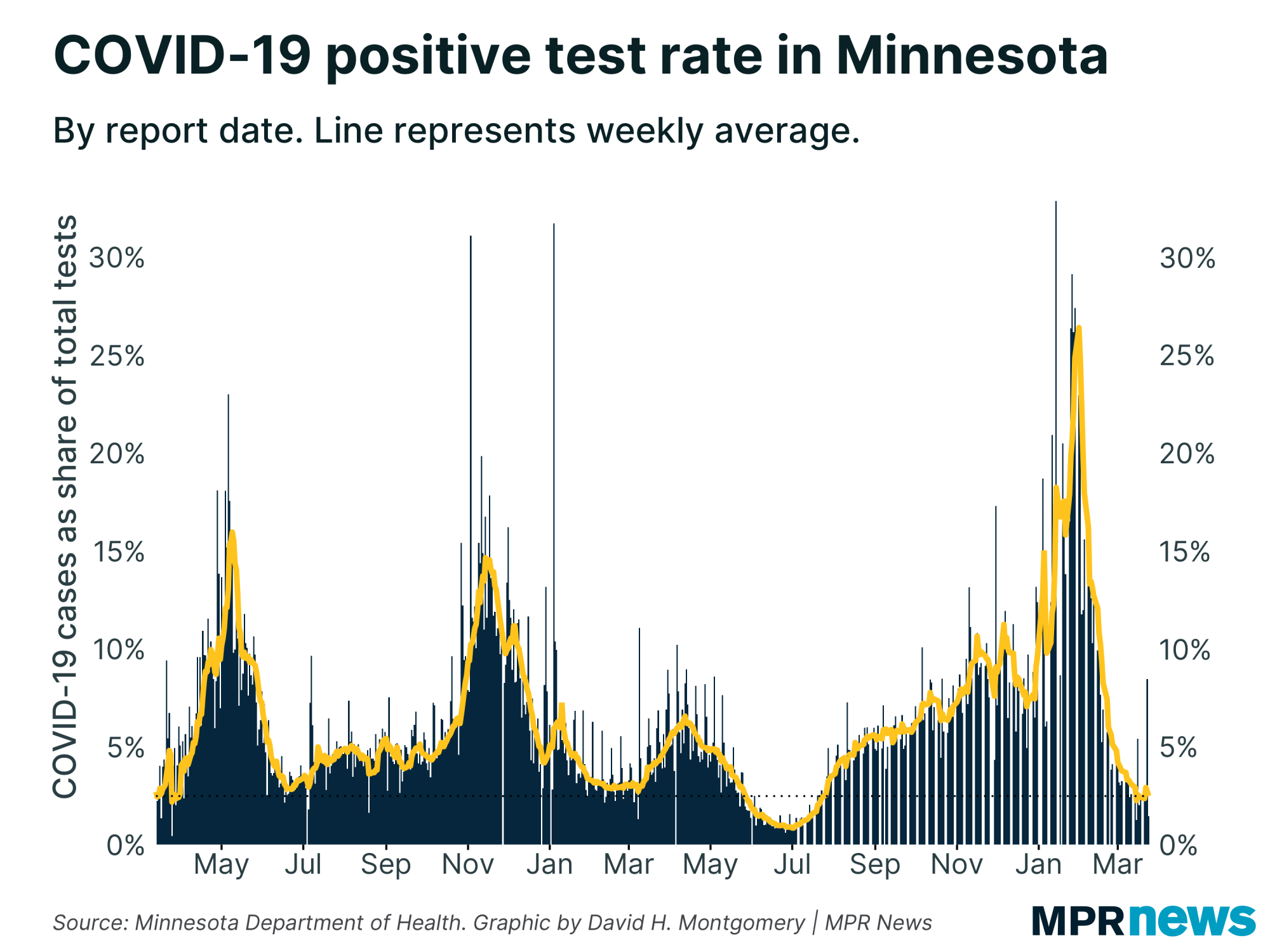
One of the most important metrics for tracking the spread of COVID-19 in Minnesota is the “positivity rate” — or how prevalent positive cases of the disease are, when compared to the number of tests being done.
In general, a low positivity rate is a good sign. Because not everyone who has COVID-19 gets tested, rising case numbers could mean that the disease is spreading, or that testing efforts are identifying a larger share of the sick people in society. Positivity rate controls for the amount of testing being done.
But calculating the positivity rate is more complicated than it might seem, because some people get tested multiple times for COVID-19.
There are two ways to calculate positivity rate:
Turn Up Your Support
MPR News helps you turn down the noise and build shared understanding. Turn up your support for this public resource and keep trusted journalism accessible to all.
Method 1: Divide (A) the number of people who have tested positive by (B) the number of people who have been tested
Method 2: Divide (C) the number of positive tests by (D) the number of total tests
These two methods won’t produce the exact same numbers, depending on how many people get tested multiple times and how many people rack up multiple positive tests. But they’ll tell the same general story.
Unfortunately, until last week, those calculations weren’t possible using the Minnesota Department of Health’s published data. The agency’s daily situation updates reported the number of people who have confirmed cases in the state (A), and the number of total COVID-19 tests (D).
Dividing the number of confirmed cases by the number of total tests wouldn’t accurately approximate the state’s positivity rate, because it’s not an apples-to-apples comparison.
One of the numbers in that equation — the number of people who tested positive (A) — was adjusted to control for the fact that some people were tested multiple times, while the other number — total tests (D) — wasn’t.
The Department of Health’s own positivity rate graphs are based on Method 2: positive tests divided by total tests. The number of positive tests each day hasn’t been included in the department’s situation updates, meaning members of the public couldn’t recreate the state’s metric.
Because the public lacked the data to properly calculate positivity rate with either Method 1 or Method 2, public calculations of Minnesota’s positivity rate, including the ones MPR News has published daily, necessarily underestimated the positivity rate.
COVID-19 math
To understand why, we need to take a brief detour back to grade-school math: Remember that a fraction consists of one number, a numerator, divided by another, a denominator.
If the denominator stays the same, then increasing the numerator will make the result larger, and decreasing it will make the result smaller: 3/8 is larger than 2/8 but smaller than 4/8.
That’s simple enough. Slightly more complicated is what happens if you keep the numerator the same and change the denominator.
For a given numerator, a smaller denominator will produce a larger number, while a larger denominator will produce a smaller one: 1/3 is larger than 1/4 but smaller than 1/2.
Now let’s see how this plays out with the positivity rate. The numerator — the number on top of the fraction — is the number of cases in Method 1, but positive tests in Method 2.
The denominator is the number of people tested in Method 1, and total tests in Method 2.
To recap:
Method 1: The number of people who have tested positive divided by the number of people who have been tested
Method 2: The number of positive tests divided by the number of total tests
Because some people get tested more than once, the number of total tests is always going to be larger than the number of people tested. The number of positive tests is going to be larger than the number of people who’ve tested positive.
On July 31, for example, Minnesota reported 779 new COVID-19 cases, and 17,034 total tests. Dividing 779 by 17,034 is 0.046, or 4.6 percent.
But some of those 17,034 tests were double-tests for individuals: Only 13,537 new people had been tested, according to the July 31 report. Dividing 779 by the number of new tests — 13,537 — equals a 5.8 percent positivity rate. That higher positivity rate is exactly what we’d expect if we keep the numerator the same and shrink the denominator.
Changing the calculation
From March 29 through June 4, the state health department released data on the total number of tests performed (D) and the total number of people tested (B).
On June 5, the department changed how it reports testing data, and began reporting total number of tests (D) instead of total number of people tested (B), while continuing to report people who had tested positive (A) rather than total number of positive tests (C).
On July 30, at the request of MPR News, the department resumed reporting the total number of people tested (B), which again allowed the public to calculate an apples-to-apples positivity rate: That’s how we were able to calculate the 5.8 percent positivity rate on July 31, compared to the less-precise 4.6 percent, calculated with the old method.
Putting this number in context is difficult, however, because the department for now is not releasing data on the number of people who were tested during that gap period, between June 5 and July 29.
Using the data available, however, it’s possible to estimate what the positivity rate would be, using the more accurate method of people who tested positive (A) divided by people who were tested (B).
In particular, because both testing figures were available before June 5 and after July 29, it’s possible to compare look at the relationship between those two data points:
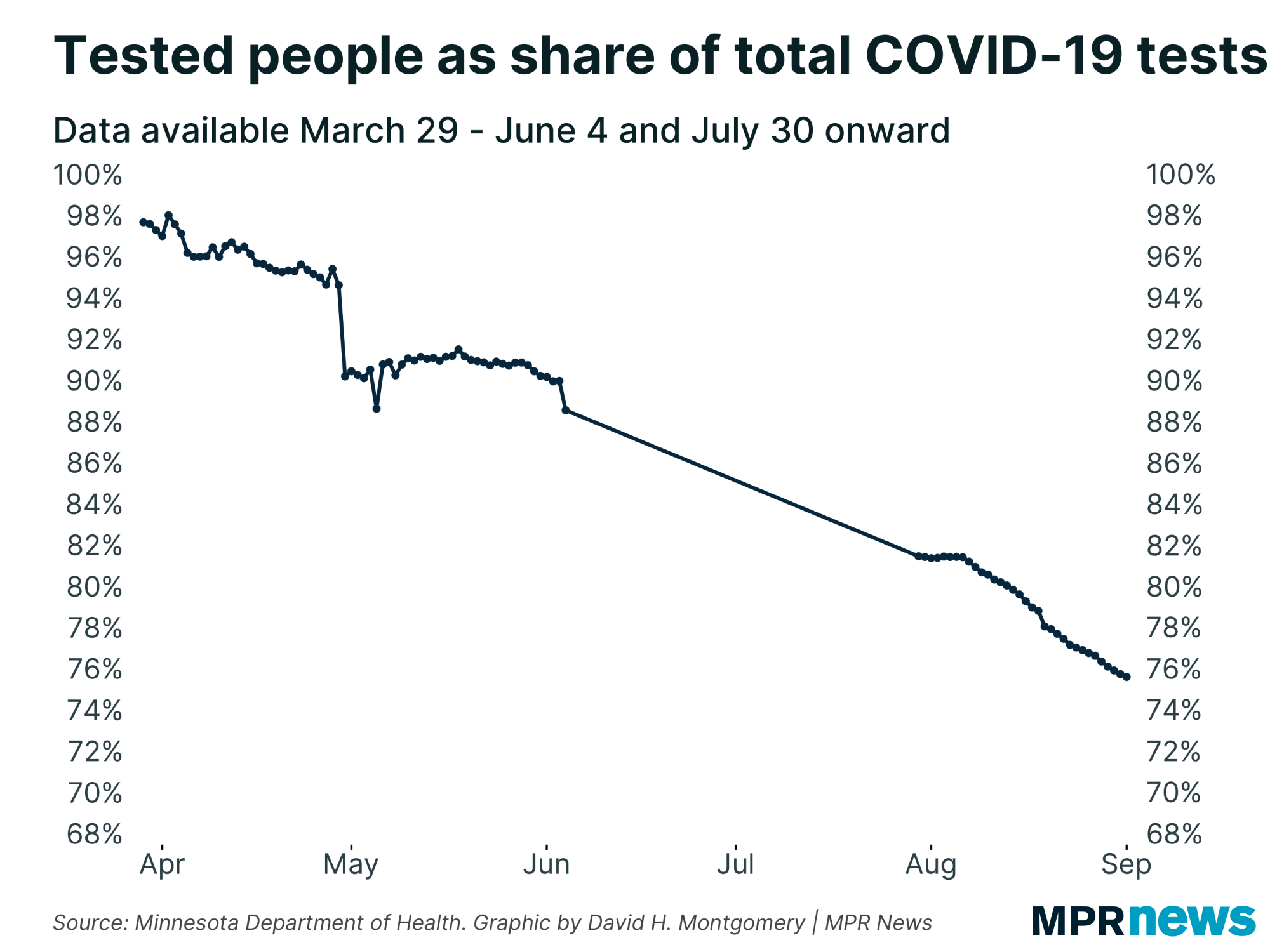
Early on in the outbreak, when tests were very limited, about 98 percent of tests were going to people who hadn’t been tested before. That number steadily dropped through April and May, before reaching 88.4 percent on June 4, the last day we had both pieces of data. When the data reappeared on June 30, this ratio had fallen to 81.6 percent.
This decline is exactly what one would expect. Even setting aside health care workers who might be tested frequently, someone who got a test in May after being exposed to COVID-19 might be exposed again in July and seek another test.
That would increase the total number of tests administered, but not the total number of people tested, and would cause the ratio between the two to decline.
In the absence of actual data, we don’t know what the ratio between total tests and total people tested looked like on each day of the gap.
But if we assume for the sake of modeling that it decreased in a linear fashion, by 0.13 percentage points per day, that can let us estimate the number of people tested each day based on that ratio and the number we do have, total tests.
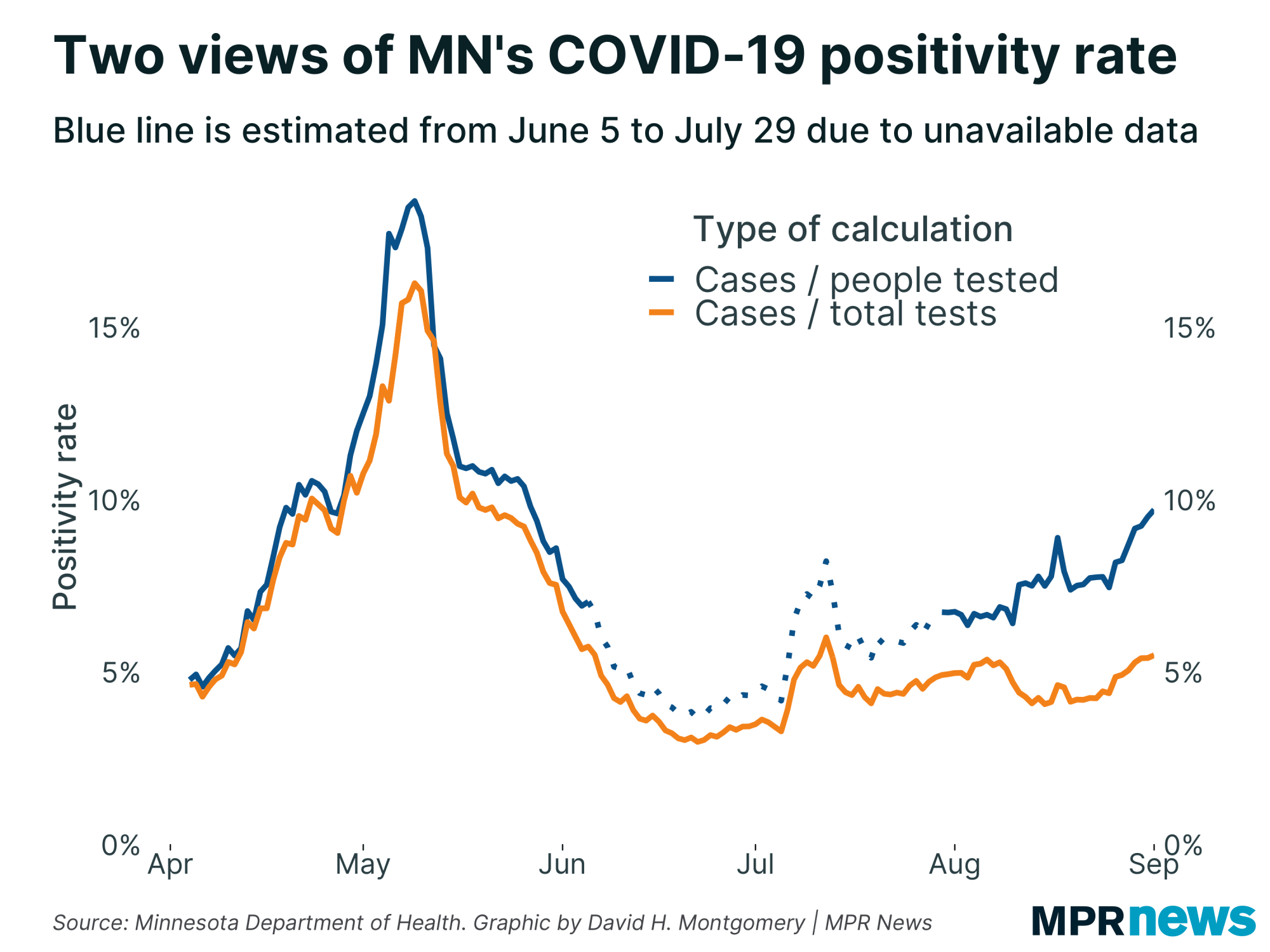
In general, this corrected data follows the same trend as the old data, just a percentage point or two higher. The COVID-19 outbreak in Minnesota, in other words, may have been a little more widespread than the earlier data made it seem.
The fact that these two lines largely move in tandem means the old data dividing cases by total tests wasn’t useless — it could still show whether the positivity rate was going up or going down.
But because the ratio between total people tested and total tests administered declines over time, the gap between these two methods of calculation will tend to expand over time.


